EN中文

地球信息科学学报 >

2020 , Vol. 22 >Issue 3: 361 - 369

DOI: https://doi.org/10.12082/dqxxkx.2020.190235

“数字地形分析”专栏

规则格网DEM局地坡面凸凹性精度分析

  • 王春 , 1, 2, * ,
  • 徐燕 1, 2 ,
  • 江岭 1, 2 ,
  • 赵明伟 1, 2
展开
  • 1. 滁州学院地理信息与旅游学院,滁州 239000
  • 2. 安徽省地理信息智能感知与服务工程实验室,滁州 239000

王 春（1975— ）,男,博士,教授,宁夏固原人,主要从事DEM数字地形分析与GIS集成应用技术研究。E-mail:wangchun93@126.com

收稿日期: 2019-05-17

  要求修回日期: 2019-08-25

  网络出版日期: 2020-05-18

基金资助

国家自然科学基金项目(41571398)

国家自然科学基金项目(41201415)

国家自然科学基金项目(41501445)

安徽省自然科学基金项目(1408085MD77)

安徽省自然科学基金项目(1608085QD77)

版权

版权所有，未经授权，不得转载、摘编本刊文章，不得使用本刊的版式设计。
收起

Accuracy Assessment of Local Slope Concave-convex Properties based on Multi-scale Gridded DEMs

  • WANG Chun , 1, 2, * ,
  • XU Yan 1, 2 ,
  • JIANG Ling 1, 2 ,
  • ZHAO Mingwei 1, 2
Expand
  • 1. Geographic Information and Tourism College ,Chuzhou University, Chuzhou 239000, China
  • 2. Anhui Engineering Laboratory of Geo-information Smart Sensing and Services, Chuzhou 239000, China
* WANG Chun, E-mail：

Received date: 2019-05-17

  Request revised date: 2019-08-25

  Online published: 2020-05-18

Supported by

National Natural Science Foundation of China(41571398)

National Natural Science Foundation of China(41201415)

National Natural Science Foundation of China(41501445)

Anhui Provincial Natural Science Foundation(1408085MD77)

Anhui Provincial Natural Science Foundation(1608085QD77)

Copyright

Copyright reserved © 2020
Fold

摘要

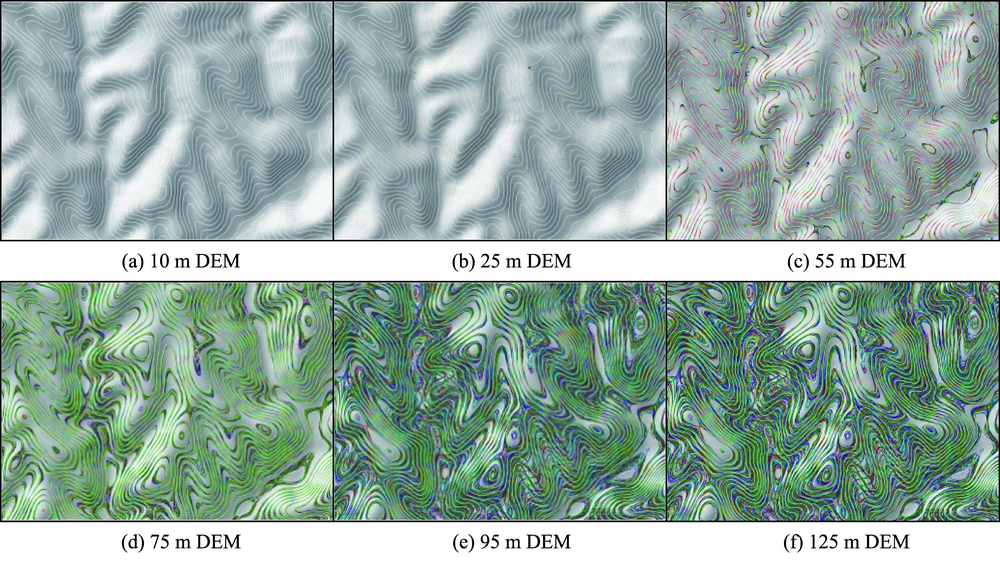
DEM地表形态精度分析理论与方法的建立,对DEM数据的生产和广泛应用具有重要意义。本文从局地坡面形态的凸凹性角度,剖析规则格网DEM格网点位置、格网分辨率对DEM局地坡面凸凹性的影响,以期进一步完善和发展DEM质量分析的理论与方法。论文首先阐述了DEM局地坡面凸凹性的基本概念,研究建立了规则格网DEM的局地坡面凸凹性量化分析方法,并以黄土丘陵5、10、15、25、……、155 m DEM为例,采用比较分析方法研究了局地坡面凸凹性随DEM格网点位置和格网分辨率的变化特征。研究表明：对于本研究中的1:5万DEM,10 m（跃变率≤ 0.3%）是其最佳的格网分辨率阈值,当DEM实际格网分辨率高于该阈值时,实际DEM与最佳格网分辨率DEM具有近乎相同的局地坡面凸凹性,主要在正地形与负地形的过渡区域会发生不同程度的坡面凸凹性变化;当DEM实际格网分辨率低于该阈值时,实际DEM的局地坡面凸凹性,会随着DEM格网点布设位置和DEM格网分辨率发生较大的不确定性变化。

关键词： DEM; 局地坡面; 凸凹性; 空间关系; 地表形态; 黄土丘陵; 误差; 精度

本文引用格式

王春 , 徐燕 , 江岭 , 赵明伟 . 规则格网DEM局地坡面凸凹性精度分析[J]. 地球信息科学学报, 2020 , 22(3) : 361 -369 . DOI: 10.12082/dqxxkx.2020.190235

Abstract

The accuracy of DEMs is the key issue for its quality and various applications. Theoretically, terrain morphology is the basis to derive the topographic information. Therefore, to establish the methodology of DEM's morphologic accuracy can promote the high-quality DEM productions and their widespread applications. Focusing on the cognition of the local slope concave-convex property, this paper analyses the influences of grid point locations and grid cell sizes on this morphological property. As such, this paper arms to further improve and develop the theory and method of DEM quality. At first, this paper introduces the basic concept of terrain morphology accuracy represented by the gridded DEM, consisting of elevation sampling accuracy and elevation relation accuracy of local points, local slope morphology accuracy, regional terrain relation accuracy and global terrain structure accuracy. Herein, the local slope morphology accuracy can be represented by the local slope concave-convex property, including three categories of flat slope, concave slope and convex slope. To quantify the concave-convex property, the error metric is defined using the multi-scale DEMs and several statistic indexes of the error metric are presented. At last, taking loess hill region as the study area and 5、10、15、25、…、155 m DEMs generated by the TIN-based method as the experimental dataset, the comparative method is applied to investigate the characteristics of the local slope concave-convex slope varied with the grid-point location and the grid cell size. The main conclusions of this research are reached as follows: (1) the morphology accuracy of DEMs must be considered in the production of high-quality DEMs. In this study, 10 m size (jumping rate is equal or lesser than 0.3%) is optimal threshold of the grid cell size for 1:50 000 DEMs. (2) When the DEM resolution is higher than the threshold, the DEM has almost the same concave-convex property with the real topography, followed by the less changes in the transition area between the positive and negative terrains. When the resolution of DEM is lower than the threshold, the local slope concave-convex property would have a significant uncertainty changed with the grid-point location and the DEM resolution.

Key words： DEM; local slope; concave-convex; spatial relationship; terrain morphology; loess hill region; error; accuracy

1 引言

地面形态是派生地形信息的本源。数字地形建模的核心任务或目的是实现不同尺度条件下地面形态的高保真模拟。然而,当前DEM的质量分析主要关注于DEM的高程数值精度[1,2,3,4],现有DEM质量标准与作业规范,都注重对DEM高程数值误差的解释和控制,相对忽视了对DEM地表形态精度的描述和说明,不仅在一定程度上导致用户无法准确推演DEM数字地形分析结果的可靠性,而且面对当前丰富的多源多尺度DEM数据,经常发生DEM数据的误用与盲从。大量研究表明,单纯依据DEM高程数据精度,无论是检查点法、传递函数法、协方差法[4,5],还是其他更为复杂的DEM误差估算模型[7,8],例如诸多学者利用地统计学方法[9]、最小二乘配置法[10]、径向基函数算法[11]、误差评价与水系提取[12]等方法作为精度评价方法,都难以具有普适性的评价实际DEM地形描述质量的空间分布情况。因此,建立DEM地表形态精度分析的理论与方法,不仅是解决DEM地形描述真实性分析与可用性评价的关键[13,14,15],同时也是规范系列尺度DEM数据生产的关键。
关于DEM地表形态精度问题,柯正谊等[16]从保凸性、逼真度、光滑性3个方面阐述了DEM地形曲面与实际地形曲面的逼近关系;汤国安等[3,17]研究提出了DEM地形描述误差概念,随后王光霞等[18]进一步完善了DEM地形描述误差的估算模型;胡鹏等[19]研究提出了DEM误差逼近论模型,提出DEM高保真要求是DEM对原高程序列的维护,并初步建立其量化分析方法;王春等[2]深入剖析了现有格网DEM地形描述的失真现象,提出了顾及地表形态精度的高保真DEM基本质量标准;一些学者[7-8,20-21]在地形综合研究中,分析DEM地形结构精度的变化特征与保真方法。相比DEM高程数值精度,DEM地表形态精度分析是更为复杂的问题,现有的研究仅仅是零散的探讨,远不足以建立完整的概念框架与量化分析方法。当前普遍采用以高程精度为分析要点的DEM精度评定方法,在本质上是把DEM理解成点栅格,没有关注相邻点之间的高低关系。众所周知,完全相同的点位高程精度,通过不断改变点位的高程误差的分布位置,可以做出无穷无尽的不同形态的DEM地形曲面,但仅有一个DEM地形曲面与实际地形曲面吻合。因此,DEM局地坡面形态的凸凹性误差虽然部分来源于DEM格网点高程误差,但与DEM格网点高程误差是完全不同的概念,DEM局地坡面形态凸凹性更强调相邻DEM格网点高程高低关系及高低程度的度量与分析,从而更好地确保DEM能准确、真实再现它所表达尺度的地表形态。
鉴于此,本文主要从局地坡面形态的凸凹性角度,剖析规则格网DEM格网点位置、格网分辨率对DEM局地坡面凸凹性的影响特征,以期进一步完善和发展DEM质量分析的理论与方法。

2 研究方法

2.1 DEM局地坡面凸凹性基本概念

DEM通过有限的规则格网采样数据实现地面形态的数字化模拟。由于数据采样误差、DEM数据结构、地表重构模型等各种因素的影响,DEM模拟地面形态与实际地面形态之间,不可避免地存在不同程度差异,本文把这种差异定义为DEM地表形态精度。由于地形表面自身以及随着尺度综合的复杂性、多样性,目前还难以用确定的数学函数实现精确表达,使得DEM地表形态精度暂不能用确定的单一数学函数表达,需要从不同的视角进行量化描述。依据“由简到繁,由表及里”原则,基于“单点→点对→坡面→区域→整体”的DEM地形表面重构过程,从单个地面点、成对地面点、局地坡面、区域地形、全局结构的角度,DEM地面形态误差可以分解为地面点高程逼近精度、地面点高低关系精度、局地坡面形态精度、区域地形关系精度和全域地形结构精度。
局部坡面形态就是指在DEM中能准确识别和提取的单一形态的坡面。研究表明,虽然不同区域的地面形态千姿百态,很难用一个确定的函数表达,但是任何复杂程度的地形,均可分解为图1所示的平直坡面、凹形坡面和凸形坡面3种单一形态坡面。对于任何尺度的DEM数据,如果能够准确记录和重构出每一个单一形态坡面,无疑该DEM已实现了地面形态的高精度描述与表达。因此,通过构建描述单一形态坡面精度的DEM局地坡面形态误差,可以解决DEM局地坡面形态精度的量化分析问题。
图1 DEM局部坡面基本形态示意

Fig. 1 Schematic diagram of DEM local slope shapes

综上所述,DEM局地坡面凸凹性误差指以地面点P为中心的局部坡面,其在DEM模拟地形中的凸、凹、平特征与其在实际地形中的凸、凹、平特征的差异。

2.2 DEM局部坡面凸凹性精度量化指标

2.2.1 局地坡面凸凹平形态的度量
图2是单一形态坡面与其一阶趋势面关系图。对于地形区域abcd,P″为区域中心点,P为对应的实际地面点,其高程记为 H p ;ABCD平直面为该区域的一阶趋势面（平直面）,P′为P″在ABCD平直面对应点的高程,其高程记为 H ˆ p 。
图2 单一形态坡面示意

Fig. 2 Illustration of slope with a single morphology

显然,对于平直面,P´与P必然重合,此时 H p = H ˆ p ;对于凸形面,P点必然在P´点上方,此时 H p > H ˆ p ;对于凹形面,P点必然在P´点下方,此时 H p < H ˆ p 。
依据 H p 和 H ˆ p 的大小关系,可建立以地面点P为中心的局地坡面凸凹性指标。凸凹性指标公式为：
TA X p = Sign H p - H ˆ p = 1 ( 凸形坡面 , H p > H ˆ p ) 0 ( 平直坡面 , H p = H ˆ p ) - 1 ( 凹形坡面 , H p < H ˆ p )
在实际应用中为了简化计算,取DEM 3 m×3 m分析窗口中DEM格网点的平均高程代替 H ˆ p 。
2.2.2 DEM局地坡面凸凹性误差度量指标
DEM局地坡面凸凹性误差度量指标计算公式为：
E tax _ p = TA X p ˆ - TA X p = { 2,1 , 0 , - 1 , - 2 }
式中： TA X p ˆ 和 TA X p 分别为以地面点P为中心的局部坡面在DEM模拟地形表面和实际地形表面中局地凸凹性指标。 TA X p 通常可以采用实地采样法或基于更高分辨率DEM数据获取,获取时需要注意每次至少采样9个以上格网点,格网点的布置近似为3×3布局。本文重点分析DEM格网大小对地表形态精度的影响特征,因此直接以原始5 m DEM为参考真值计算出 TA X p ,据此分析10、15、25、35、…、155 m DEM地表形态精度的变化特征。式（2）计算结果的含义如表1所示。
表1 Etax_P值含义

Tab. 1 Means of Etax_P

Etax_P值 代表含义
2 局地坡面形态由原来的凹形坡面变为凸形坡面
1 局地坡面形态由原来的凹形坡面变成平直坡面,或由原来的平直坡面变成凸形坡面
0 局地坡面形态没有变化
-1 局地坡面形态由原来的凸形坡面变成平直坡面,或由原来的平直坡面变成凹形坡面
-2 局地坡面形态由原来的凸形坡面变为凹形坡面
2.2.3 DEM局地坡面形态的精度指标
依据DEM局地坡面形态凸凹误差的性质特征,论文研究建立了表2所示的精度指标,以揭示其数值分布规律。
表2 DEM局地坡面形态凸凹性精度指标信息

Tab. 2 Concave and convex accuracy indexes of local slope shapes

序号 指标名称 计算公式 指标含义 公式编号
1 算数平均值 Ex̅=∑i=1nEtax_in 所有检测点凸凹性误差的平均值。主要反映与局地坡面形态凸凹性误差是否存在系统规律。Etax_i含义见表1,n为总检测点个数 （3）
2 正确率值 Ex_0=k0n×100 k0是所有检测点中凸凹性未发生改变的坡面个数,n为总检测点个数 （4）
3 渐变率值 Ex_1=k1n×100 k1是所有检测点中按照“凸平凹或凹平凸”次序,发生凸变平、平变凹、凹变平或平变凸等变化的坡面个数,n为总检测点个数 （5）
4 跃变率值 Ex_2=k2n×100 k2是所有检测点中从“凸平凹或凹平凸”次序,发生凸变凹或凹变凸变化的坡面个数,n为总检测点个数 （6）

3 DEM局地坡面形态精度实验分析

3.1 实验样区与实验数据

实验样区位于陕西省绥德县无定河中游韭园沟,海拔814~1188 m,相对高差374 m,地面平均坡度约28.6º,区内丘陵起伏,沟壑纵横,土壤侵蚀极为剧烈,地面形态类型复杂,属于黄土丘陵沟壑区地貌特征。图3为实验样区叠加等高线后的1:5万DEM地形晕渲图。
图3 实验样区5 m DEM地形晕渲

Fig. 3 Hillshade of 5 m DEM in test area

实验数据的制作过程为：
（1）参照国家由地形图生产格网DEM的技术流程,首先基于矢量化的1:5万地形图中的等高线、高程点、水系等数据,采用ArcGIS 9.3中的“Create TIN from features”工具,生成实验样区初始TIN模型;
（2）采用人机交互方式消除伪平三角,形成不含伪平三角的正确TIN模型;
（3）采用ArcGIS 9.3中的“TIN to Raster”工具,首先生成1 m DEM作为基础数据,取5 m DEM作为参考真值DEM,记为DEM5;然后采用不断变换DEM格网点位置的方法,从1 m DEM中分别得到不同格网点布设位置的10、15、25、35、……、155 m DEM各25幅,记为DEMk_i。k为DEM格网分辨率值,取值分别为10、15、25、35、……、155;i为不同格网点布设位置的DEM标识码,取值分别为1、2、3、……、25 。

3.2 实验分析方法与流程

严格意义上的局地坡面形态误差计算,需要分别以DEM5、DEM15_i、DEM25_i、DEM55_i、DEM75_i、DEM125_i数据为基础,建立严密的三维数学曲面方程,然后逐点计算每个检测点的凸凹度和凸凹性误差。由于地面形态的复杂多样性,目前的DEM地面形态重构过程中,难以给出具有明确表达式的全域DEM地形曲面方程,通常采用DEM格网加密方法计算DEM地形曲面高程。研究发现,采用以 4 d×4 d分析窗口（d为DEM格网分辨率大小）为搜索圆的完全规则样条函数插值法,所重构的DEM地面形态最优[13,22],据此本文设计了如下实验分析过程：
（1）采用上述完全规则样条函数插值法,对DEMk_i进行DEM格网加密处理,生成与DEM5具有相同格网属性（格网大小和格网点位置）的DEM,记为nDEMk_i;
（2）分别计算DEM5、nDEMk_i的局地坡面形态误差值,记为TAX5和TAXk_i;
（3）计算TAXk_i与TAX5的差值,获得对应的凸凹度误差场,记为Etax_ki,然后计算表2指标并分析其变化特征。
DEM格网点高程误差的变化,利用该DEM生成的等高线,在该DEM格网点区域必然会发生形态或位置的变化。因此,本文利用等高线套合,能从视觉上形象、直观地反映DEM地形表达是否一致及变化特征,从而在一定程度映照本文局地坡面凸凹性的变化特征。
图4是nDEM10_i、nDEM25_i、nDEM55_i、nDEM75_i、nDEM95_i、nDEM125_i的10 m等高线（彩色线）自套合图及其与DEM5 10 m等高线（红色线）的套合图。图4（a）、图4（b）表明,10 m、25 m DEM虽然格网分辨率和格网点布设位置不同,但是经格网加密处理后,生成的等高线与原始5 m DEM等高线严密重合,即经格网加密处理后的10 m、25 m DEM,均能准确唯一地再现出了原始5 m DEM的地面形态,地形描述质量没有受到DEM格网分辨率和格网点布设位置的影响。
图4 不同格网分辨率DEM等高线套合误差分布

Fig. 4 Spatial distribution of contour register differences under different DEM cell sizes

图4（c）-（f）表明,55、75、95和125 m DEM经格网加密处理后,山脊、山谷、坡脚等地形变化比较丰富的地形单元,不仅其所生成的等高线和原始等高线存在较大误差,而且会随着实际DEM格网点布设位置的变化而发生不确定性变化,难以准确稳定地再现原始地形。

3.3 局地坡面凸凹性误差变化特征分析

图5是10、25、55、75、125 m DEM局地坡面凸凹性的误差平均值、正确率值、渐变率值、跃变率值的空间分布图。图5显示,10 m与25 m,75 m与125 m DEM局地坡面凸凹性误差平均值、正确率值、渐变率值、跃变率值的空间分布类似。此外,对比分析本实验中10~155 m DEM所对应的局地坡面凸凹性误差分布图也发现,10~25 m DEM局地坡面凸凹性误差分布图具有较好地相似性,都是在地形凸凹变化部位出现误差分布,且误差分布随着DEM格网分辨率的降低而逐渐增强;30~155 m DEM局地坡面凸凹性误差分布图具有较好地相似性,其变化特征主要体现在2个方面。① 与10~25 m DEM局地坡面凸凹性误差分布图相比,其局地坡面凸凹性误差出现部分宽度较小或凸凹程度较小的单一凸形坡和凹形坡部位,且出现的面积随着DEM格网分辨率的降低而迅速扩大;② 在地形凸凹变化部位出现误差的显著程度随着DEM格网分辨率的降低迅速扩大。
图5 DEM局地坡面凸凹性的误差平均值、正确率值、渐变率值、跃变率值的空间分布

Fig. 5 Spatial distribution of concave and convex accuracy indexes of DEM local slope shapes

图5表明,DEM局地坡面凸凹性误差的平均值、正确率、渐变率和突变率呈现出明显的结构性分布特征,其中：
（1）在10 m和25 m DEM中,DEM局地坡面凸凹性误差主要出现在沟沿线等地形变化部位,其他的地形部位基本没有出现DEM局地坡面凸凹性误差。也就是说,在10 m和25 m DEM中,DEM局地坡面凸凹性主要和DEM格网分辨率有关,DEM格网点具体布设位置对其没有影响,DEM地形描述结果具有很好稳定性和唯一性。
（2）在55、75和125 m DEM中,DEM局地坡面凸凹性误差不仅主要分布在沟沿线等地形变化部位,在其它地形部位均有不同程度的分布,而且随着DEM格网分辨率的降低,DEM局地坡面凸凹性误差的结构性分布及全坡面分布的特征也迅速增加。也就是说,此时的DEM中,DEM局地坡面凸凹性和DEM格网分辨率大小及格网点实际布设位置密切相关,DEM地形描述结果不具有稳定性和唯一性。
表3是10、15、25、35、……、155 m 等不同格网分辨率DEM（每个格网分辨率均有25幅不同格网点布设位置的DEM）,以每一幅DEM为统计对象的局地坡面形态凸凹性误差的统计信息。表中记录形式为K±m,K为25幅不同格网点布设位置的DEM,其不同类型局地坡面形态凸凹性精度指标的平均数,m为其最大波动范围。
表3 DEM局地坡面凸凹性精度指标

Tab. 3 Concave and convex accuracy indexes of DEM local slope shapes

DEM格网
分辨率/m		 正确率/% 渐变率/% 跃变率/%
凹变平或平变凸 凸变平或平变凹 合计 凹变凸 凸变凹 合计
10 96.81±0.0236 1.55±0.0119 1.50±0.0178 3.05±0.0222 0.08±0.0019 0.07±0.0024 0.14±0.0033
15 94.66±0.0192 2.37±0.0169 2.22±0.0165 4.58±0.0222 0.38±0.0077 0.38±0.0079 0.76±0.0090
25 90.88±0.0263 3.09±0.0211 2.84±0.0308 5.93±0.0248 1.59±0.0177 1.50±0.0185 2.98±0.0206
35 87.41±0.0367 3.41±0.0257 3.12±0.0218 6.53±0.0313 3.14±0.0173 2.92±0.0215 6.06±0.0216
45 83.42±0.0600 3.58±0.0218 3.29±0.0255 6.87±0.0275 5.07±0.0281 4.65±0.0392 9.72±0.0563
55 79.42±0.0986 3.59±0.0259 3.35±0.0272 6.94±0.0314 7.08±0.0401 6.56±0.0937 13.63±0.1027
65 75.43±0.1292 3.61±0.0317 3.41±0.0298 7.02±0.0378 9.08±0.0557 8.47±0.1200 17.55±0.1151
75 71.96±0.1499 3.56±0.0235 3.42±0.0197 6.99±0.0333 10.85±0.0976 10.20±0.0613 21.05±0.1409
85 70.14±0.1951 3.63±0.0413 3.50±0.0383 7.12±0.0547 11.67±0.1313 11.07±0.0563 22.74±0.1696
95 68.62±0.1023 3.78±0.0464 3.62±0.0264 7.40±0.0426 12.22±0.0958 11.76±0.0690 23.98±0.1183
105 66.18±0.1775 3.86±0.0386 3.70±0.0305 7.56±0.0427 13.36±0.1314 12.89±0.1080 26.26±0.1451
115 65.57±0.1695 4.19±0.0478 3.99±0.0495 8.18±0.0527 13.28±0.1289 12.97±0.0820 26.25±0.1567
125 64.68±0.3153 4.72±0.0789 4.48±0.0634 9.20±0.1017 13.15±0.2207 12.98±0.1479 26.12±0.2512
135 61.91±0.3243 4.58±0.0507 4.41±0.0509 8.99±0.0763 14.73±0.2135 14.38±0.1222 29.11±0.2769
145 61.05±0.2989 5.17±0.0954 4.94±0.1247 10.11±0.2015 14.52±0.1501 14.33±0.1521 28.85±0.2212
155 59.29±0.5449 5.44±0.1399 5.22±0.1362 10.65±0.2680 15.17±0.2515 14.88±0.1730 30.05±0.3604

注：表中记录形式为K±m; k为25幅不同格网点布设位置的DEM,其不同类型局地坡面形态凸凹性精度指标的平均数;m为其最大波动范围。

图6为实验样区DEM局地坡面凸凹性的正确率值、渐变率值、跃变率值的概率分布图及其随DEM格网分辨率的变化曲线图。
图6 验样区DEM局地坡面凸凹性的正确率值、渐变率值、跃变率值变化曲线

Fig. 6 Spatial distribution of maximum and minimum of elevation numerical error

结合图6和表3数值,对于本文中的1:5万黄土丘陵地形,可以得出：
（1）10、15 m DEM虽然具有不同的格网分辨率值,但具有同等数量级的局地坡面凸凹度精度,即10 m DEM仅增加了DEM数据冗余,并未有效提高DEM局地坡面凸凹性正确率精度,但10 m DEM的局地坡面凸凹性跃变率精度比较理想,能稳定地控制在0.3%以内,在一定程度可以保证DEM地形描述不会出现形态粗差。
（2）目前的国家规范将1:5万DEM格网分辨率设置为25 m。本文实验表明,在黄土丘陵地区,我国当前的25 m格网分辨率DEM,其局地坡面凸凹性正确率能达到90%以上,但跃变误差也接近3%。也就是说,我国黄土丘陵地区当前的25 m格网分辨率DEM,能够很好反映大多数区域的局地形态特征,但地形起伏变化丰富部位的局地形态凸凹精度较差。
（3）对于本文黄土丘陵地区的1:5万DEM,如果DEM格网分辨率低于25 m,DEM地形描述的凸凹形态精度不仅随着DEM格网分辨率的降低而降低,而且会随着DEM格网点实际布设位置的不同而不同,将很难有效保证DEM局地坡面凸凹性,此时的DEM将难以准确、稳定再现原始地形的坡面凸凹形态。

4 结论与讨论

4.1 结论

（1）DEM高程数值精度和地表形态精度是有联系,但更有区别的概念。现有的高程精度计算方法,并不考虑DEM地面点之间的高程高低关系,致使即便是同一地区的同样高程精度的DEM,可以有无穷多的地表形态[2-3,13],高精度DEM的建设必须考虑地表形态精度问题。本文研究显示,对于1:5万黄土丘陵地区DEM,顾及地表形态凸凹性且要求其跃变率≤0.3%时（即出现凸凹性跃变误差的概率为0.3%,出现时可按照粗差进行核检）,其最佳格网分辨率应为10 m,即图面0.2 mm DEM。
（2）对于本文黄土丘陵1:5万DEM,当其格网分辨率高于10 m时,虽然二者具有不同的格网分辨率值,但实际DEM与10 m DEM具有近乎相同的局地坡面凸凹性,仅在凸凹坡面转折部位,即正地形与负地形的过渡区范围,会发生不同程度的坡面凸凹性变化,此时提高DEM格网分辨率,仅会大幅度增加DEM数据冗余,而不会同比例提升局地坡面凸凹性精度。
（3）对于本文黄土丘陵1:5万DEM,当其格网分辨率低于10 m时,虽然局地坡面凸凹性渐变率没有发生较大变化,但是突变率会急剧增大,正确率会急剧降低,即便是相同格网分辨率DEM,局地坡面凸凹性还会随着DEM格网点实际布设位置的不同而不同,DEM地形描述结果不具有稳定性和唯一性,此时的DEM已不能准确再现原始地形的局地坡面形态。

4.2 讨论

（1）高精度或高保真DEM构建是实现高精度数字地形分析和地表过程模拟的基础,实现完整系统的DEM地表形态精度分析具有重要意义。本文仅研究了1:5万黄土丘陵地区DEM局地地表形态凸凹性,关于其他区域和比例尺DEM的局地地表形态凸凹度精度、局地地表形态凸凹性、凸凹度精度与DEM高程数值精度、局地形态复杂度等具有怎样的对应关系,对地形参数提取、地表过程模拟等具有这样的影响特征等,还有待于进一步深入研究。
（2）本文研究得出对于黄土丘陵1:5万 DEM,顾及地形凸凹形态精度时,其最佳格网分辨率应为10 m,在1:5万地形图中实地10 m也就是纸质图面的0.2 mm,正好是人对纸质图面的裸眼分辨率。因此,如果要让DEM的地表形态与同比例尺地形图对应,其最佳格网分辨率应该取对应比例尺纸质图面的0.2 mm。这一推测是否具有普适性,有必要进行系列实验分析。

参考文献

原文顺序 | 文献年度倒序 | 文中引用次数倒序
[1]
胡海, 吴艳兰, 胡鹏 . 数字高程模型精度标准、质量理论和科学观念讨论[J]. 武汉大学学报·信息科学版, 2011,36(6):713-716.

[ Hu H, Wu Y L, Hu P . Discussion of DEM standards, quality theory and conceptions[J]. Geomatics and Information Science of Wuhan University, 2011,36(6):713-716. ]

[2]
王春, 刘学军, 汤国安 , 等. 格网DEM地形模拟的形态保真度研究[J]. 武汉大学学报·信息科学版, 2009,34(2):146-149.

[ Wang C, Liu X J, Tang G A , et al. Morphologic fidelity of grid digital elevation model[J]. Geomatics and Information Science of Wuhan University, 2009,34(2):146-149. ]

[3]
王春, 汤国安, 戴仕宝 , 等. DEM地形描述误差场量化分析研究[J]. 武汉大学学报·信息科学版, 2014,39(9):1074-1079.

[ Wang C, Tang G A, Dai S B , et al. Quantitative analysis of DEM terrain representation error field[J]. Geomatics and Information Science of Wuhan University, 2014,39(9):1074-1079. ]

[4]
汤国安, 李发源, 刘学军 . 数字高程模型教程[M]. 北京: 科学出版社, 2010.

[ Tang G A, Li F Y, Liu X J. Digital elevation model course[M]. Beijing: Science Press, 2010. ]

[5]
李志林, 朱庆 . 数字高程模型[M]. 武汉: 武汉大学出版社, 2000.

[ Li Z L, Zhu Q. Digital elevation model[M]. Wuhan: Wuhan University Press, 2000. ]

[6]
Hutchinson M F . A new procedure for girding elevation and stream line data with automatic removal of spurious pits[J]. Journal of Hydrology, 1989,106(3/4):211-232.

[7]
Li, Z. and Openshaw S. A natural principle for the objective generalization of digital maps[J]. Cartography and Geographic Information Systems, 1993,20(1):19-29.

[8]
Kraus K, Otepka J. DTM modelling and visualization-The SCOP approach[C]. Photogrammetric Week, Heidelberg: Wichmann Verlag, 2005, 241-252.

[9]
宋向阳, 吴发启 . 几种插值方法在微DEM构建中的应用[J]. 水土保持研究, 2010,17(5):45-50.

[ Song X Y, Wu F Q . Application of the spatial interpolation methods to the study on micro-DEM[J]. Research of Soil and Water Conservation, 2010,17(5):45-50. ]

[10]
陈传法, 蔡乾广 . DEM快速构建的最小二乘配置法[J]. 武汉大学学报·信息科学版, 2013,38(1):86-89.

[ Chen C F, Cai Q G . High speed DEM construction based on local least squares collocation[J]. Geomatics and Information Science of Wuhan University, 2013,38(1):86-89. ]

[11]
张锦明, 游雄, 万刚 . 径向基函数算法中插值参数对DEM精度的影响[J]. 武汉大学学报·信息科学版, 2013,38(5):608-612.

[ Zhang J M, You X, Wan G . Effects of interpolation parameters in multi-log radial basis function on DEM accuracy[J]. Geomatics and Information Science of Wuhan University, 2013,38(5):608-612. ]

[12]
张彩霞, 杨勤科, 段建军 . 高分辨率数字高程模型的构建方法[J]. 水利学报, 2006,37(8):1009-1014.

[ Zhang C X, Yang Q K, Duan J J . Method for establishing high resolution digital elevation model[J]. Journal of Hydraulic Engineering, 2006,37(8):1009-1014. ]

[13]
王春, 江岭, 徐静 , 等. DEM地面形态重构方法的精度差异特征研究[J]. 地理与地理信息科学, 2014,30(4):18-21,26.

[ Wang C, Jiang L, Xu J , et al. Accuracy differences among approaches of DEM surface morphology reconstruction[J]. Geography and Geo-information Science, 2014,30(4):18-21,26. ]

[14]
薛凯凯, 熊礼阳, 祝士杰 , 等. 基于DEM的黄土崾岘提取及其地形特征分析[J]. 地球信息科学学报, 2018,20(12):1710-1720.

[ Xue K K, Xiong L Y, Zhu S J , et al. Extraction of loess dissected saddle and its terrain analysis by using digital elevation models[J]. Geography and Geo-information Science, 2018,20(12):1710-1720. ]

[15]
王春, 顾留碗, 陶旸 , 等. DEM地形描述误差(Et)计算模型研究[J]. 地球信息科学学报, 2014,16(5):699-706.

[ Wang C, Gu L W, Tang Y , et al. A new calculation model of DEM terrain description error[J]. Journal of Geo-information Science, 2014,16(5):699-706. ]

[16]
柯正谊, 何建邦, 池天河 . 数字地面模型[M]. 北京: 科学出版社, 1993.

[ Ke Z Y, He J B, Chi T H. Digital terrain model[M]. Beijing: Science Press, 1993. ]

[17]
Tang G A . A sensitivity analysis of errors in digital terrain models[D]. Salzburg: Salzburg University, 1998.

[18]
王光霞, 朱长青, 史文中 , 等. 数字高程模型地形描述精度的研究[J]. 测绘学报, 2004,33(2):168-173.

[ Wang G X, Zhu C Q, Shi W Z , et al. The further study on the accuracy of DEM terrain representation[J]. Acta Geodaetica et Cartographica Sinica, 2004,33(2):168-173. ]

[19]
胡鹏, 杨传勇, 吴艳兰 , 等. 新数字高程模型–理论、方法、标准和应用[M] 北京: 测绘出版社, 2007.

[ Hu P, Yang C Y, Wu Y L , et al. New DEM theories methods standards and application[M]. Beijing: Surveying and Mapping Press, 2007. ]

[20]
Zhang L, Li N, Jia S , et al. A method for DEM construction considering the features in Intertidal zones[J]. Marine Geodesy, 2015,38(2):163-175.

DOI PMID

[21]
Zhou B, Xu J, Zhang X , et al. New high-fidelity terrain modeling method constrained by terrain semanteme[J]. PLoS ONE, 2018,13(6):e0198530.

DOI PMID

[22]
王春, 李虎, 杨军生 , 等. 新型格网DEM等高线生成技术与方法[J]. 地球信息科学学报, 2015,17(2):160-165.

[ Wang C, Li H, Yang J C , et al. Study on generation technique of high quality contour lines based on grid DEM[J]. Journal of Geo-information Science, 2015,17(2):160-165. ]

Options
文章导航

/

〈 〉