EN中文

Journal of Geo-information Science >

2021 , Vol. 23 >Issue 2: 307 - 317

DOI: https://doi.org/10.12082/dqxxkx.2021.200189

Periodic Optimization of Drug Purchase Needs on Site for Chronic Diseases under the Epidemic Prevention and Control

  • WANG Xiaofan ,
  • FANG Zhixiang , * ,
  • ZHONG Haoyu ,
  • ZOU Xinyan
Expand
  • State Key Laboratory of Information Engineering in Surveying, Mapping and Remote Sensing, Wuhan University, Wuhan 430000, China
* FANG Zhixiang, E-mail:

Received date: 2020-04-22

  Revised date: 2020-07-06

  Online published: 2021-04-25

Supported by

National key research and development program of China(2017YFB0503802)

the Fundamental Research Funds for the Central Universities(2042020kfxg24)

Copyright

Copyright reserved © 2021.
Fold

Abstract

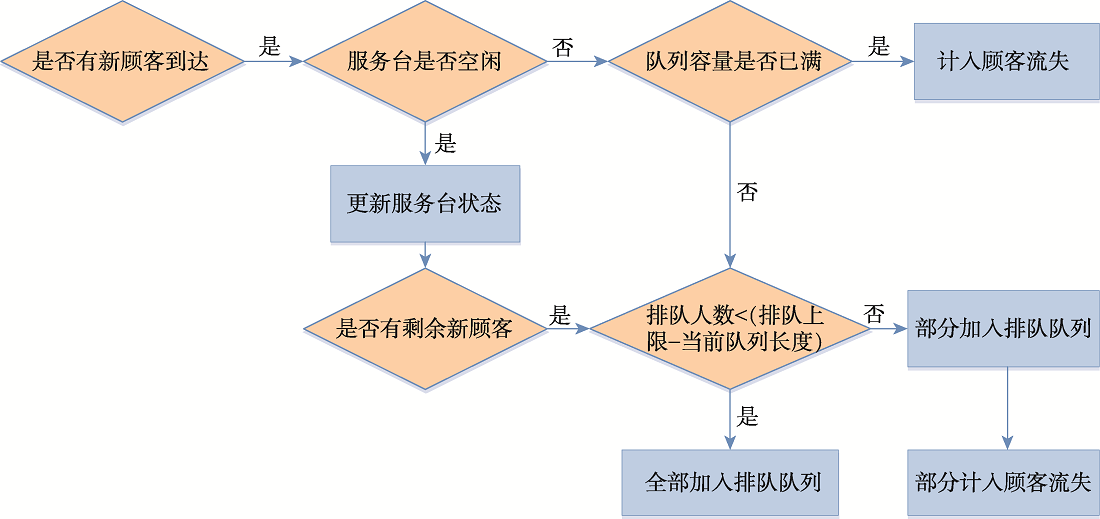
The spread of respiratory diseases among people is likely to cause large-scale public health events. During the epidemic prevention and control period, the risk of infection caused by the accumulation of drug purchase can be effectively controlled and reduced by restricting the time cycle of drug purchase. This paper focuses on the drug purchase needs of patients with chronic diseases at the early stage of closure and resumption of work in the epidemic prevention and control period and proposes an optimization method for the time cycle of drug purchase according to the scene in the drugstore. First of all, according to the principle of "no drug purchase across districts" during the epidemic prevention and control period in Wuhan, the possible service relationship between the drugstore and the community was constructed through a complex network model. Then, based on the order data of Shared bikes, the attenuation law of the drug flow along with the distance was extracted, and on this basis, the distribution mechanism of the drug flow in the community was established among the drugstores providing services for it, so as to determine the customer arrival rate at the pharmacy side and realize the coupling of the spatial distribution characteristics of the community and the pharmacy with the queuing theory model. Additionally, the discrete event simulation method was used to simulate the system state under different time cycles of drug purchase. Finally, based on the simulation results, the optimal time cycle was found by using dichotomy to maximize the consideration of patients' demands for drugs and the utilization rate of drugstores. In our study, we took Wuhan central city as an example. First, the number of patients with various chronic diseases was estimated through conditional probability, and then the optimal drug purchase cycle was obtained by using the above method for 28 days. Under the optimal time cycle, the patient's average waiting time was 8.55 min, close to the optimal duration of waiting, and the vast majority of purchasing medicine needs can be met. Also, drugstores can be fully used (More than 87% of drugstores were busy), and the daily stock quantity of drugstores was not more than 100 (bottle or box), which was within the drugstore's capacity. The methods and conclusions of this paper can be used to guide the purchasing decisions of patients with chronic diseases and the preparation of chronic diseases in drugstores.

Key words： respiratory infections; chronic diseases; queuing theory; complex network; discrete event based simulation; dichotomy; cycle; optimization

Cite this article

WANG Xiaofan , FANG Zhixiang , ZHONG Haoyu , ZOU Xinyan . Periodic Optimization of Drug Purchase Needs on Site for Chronic Diseases under the Epidemic Prevention and Control[J]. Journal of Geo-information Science, 2021 , 23(2) : 307 -317 . DOI: 10.12082/dqxxkx.2021.200189

1 引言

呼吸道传染性疾病是指病原体从人体的鼻腔、咽喉、气管和支气管等部位入侵后引起的具有传染性的疾病[1],此类疾病容易造成公众感染率的急骤升高[2],进而形成重大的公共卫生事件,严重影响居民的日常生活和经济社会的正常运转,如2003年发生的非典型性肺炎（SARS）与2019年全球新型冠状病毒肺炎（COVID-19）。在疫情防控期间和复工复产[3]初期急需保障高血压、糖尿病、冠心病等慢性病患者的购药和用药需求,否则容易引起病情恶化。一方面这类患者对药品具有长期的依赖性[4],另一方面慢性病患者人数众多,已成为我国居民重大死因疾病之一[5]。因此,研究保障慢性病药品的供应具有重大现实意义。由于疫情防控和复工复产期间人群流动仍受到严格限制,需要设定科学合理的购药周期,一方面尽可能减少人均购药次数、降低感染风险,另一方面有利于调节药品供应链的压力,实现药品的精准供给。
确定最优购药周期的基本立足点是保障药品的供需平衡。在供需平衡模型的相关研究中,价格对供需关系的调整作用是一个研究热点,有学者采用模糊模型分别建立了价格与供应和需求之间的非线性关系,通过联立求解得到了供求平衡价格的有效解[6]。还有学者从市场的作用机理出发,通过系统动力学模型对供给和需求之间的动态反馈关系进行建模[7]。此外,探究某种因素对供需平衡的影响有助于深化对系统作用机理的认知,有学者通过蒙特卡洛仿真方法模拟了天然气市场价格变动对供给和需求量的影响[11]。疫情防控下的药品供需平衡是一种较为特殊的情况,不能仅依靠价格和市场的调节作用实现供需平衡,而是需要权衡供应能力和需求量,在一定的约束条件下,求解最优购药周期,人为地调控供需均衡性。
本文假设药品供应充足,以减少购药次数并充分利用药店资源为切入点,优化购药周期。在到店场景下,购药周期直接影响药店的服务压力、药店与小区间的供需均衡性。该影响机制同小区与药店的空间分布特征密切相关,因此需结合空间数据量化这种影响关系。目前,排队论是量化服务台服务压力的常用方法,该模型能够依据服务台的顾客到达速率和服务速率计算反映服务压力的相关指标,如平均队列长度和人均等候时长[9]。但该方法无法有效考虑空间布局差异性等因素对建模结果的影响,而且使用该模型需以服务台达到稳态为前提,即顾客到达速率不大于服务速率,但实际情况中常常无法保障。
针对排队论的两点限制,本研究采用基于离散事件仿真的方法,对顾客排队购药的过程进行仿真,以求解对应的购药周期优化结果。仿真之前,通过构建药店与居民小区间的复杂网络,建立两者之间可能的服务关系[10],依据购药相关的共享单车订单数据,明确购药人流随距离的变化规律,在此基础上建立起小区购药人流在各药店间的分配机制,进而确定药店侧的顾客到达速率,实现空间因素与排队论的耦合,最终通过二分法查找仿真结果中未满足的购药人数从有到无的临界点,将其对应的购药周期作为最优解。

2 研究方法

为明确购药需求,首先需要对慢性病患者的患病情况进行分类,并估算各类患者的数量;然后通过复杂网络模型,构建药店与小区之间可能的服务关系,并基于共享单车订单数据提取购药人流量随距离的衰减规律,在此基础上计算小区购药人流在为其提供服务的各药店间的分配比例,进而确定药店侧的顾客到达速率,实现小区和药店的空间分布特征与排队论模型的耦合;接着依据真实的购药过程对药店侧的服务规则进行相应的设定;在此基础上,采用离散事件仿真方法对不同购药周期下的系统状态进行仿真;最后基于仿真结果,以最大程度地兼顾患者的购药需求和药店的使用率为优化目标,通过二分法查找购药周期的最优解。技术路线图如图1所示。
图1 技术路线

Fig. 1 Technology roadmap

2.1 居民慢性病患病概率估算

从病理学角度来看,患有某种慢性病的人群 比普通人有更高的概率患有另一种慢性病[8],因此,慢性病患者常同时患有多种慢性病。本文将高血压患者记为A类病人,糖尿病患者记为B类病人,冠心病患者记为C类病人,进一步将慢性病患病情况细分为7类,各类情况间的概率关系见 式（1）—式（7）,其中涉及的已知参数取值见表1。
P ( A ) = P A ̅ + P ( AB ) + P ( AC ) - P ( ABC )
P ( B ) = P B ̅ + P ( AB ) + P ( BC ) - P ( ABC )
P ( C ) = P C ̅ + P ( AC ) + P ( BC ) - P ( ABC )
P ( AB ) = P ( A | B ) × P ( B )
P ( BC ) = P ( C | B ) × P ( B )
P ( AC ) = P ( A | C ) × P ( C )
P A ⋃ B ⋃ C = P A + P B + P C - P AB - P BC - P AC + P ABC
表1 式（1）—式（7）中已知参数取值

Tab. 1 Parameters are known in equation (1)-(7)

参数 含义 取值
P(A⋃B⋃C) 患三类病 0.2912[12]
P(A) 患高血压 0.2145[12]
P(B) 患糖尿病 0.1050[13]
P(C) 患冠心病 0.0540[14]
P(A|B) 高血压患者患有糖尿病 0.4000[15]
P(A|C) 高血压患者患有冠心病 0.7000[16]
P(C|B) 冠心病患者患有糖尿病 0.1670[17]
高血压、糖尿病、冠心病属于重症慢性疾病,一般情况下,这类患者需要通过门诊诊断,再由医生 开具处方,凭处方购药。在疫情防控期间,为保障 患者用药,湖北省卫建委推出了慢性病长处方制度,即在医生许可下,患者在一定期限内（12~14周）,可凭旧处方前往药店购药。经调查了解,治疗高血压、糖尿病、冠心病的药品在一定规模的连锁药店均有销售。因此,本文假设疫情防控和复工复产期间,连锁药店可为市民提供购药服务。

2.2 药店与居民小区间服务人流分配

通过构建药店与居民小区间的复杂网络,来确定药店与小区间的服务关系。该网络包括连接关系及流量分配。连接关系的建立参考了疫情期间武汉市购药政策——市民不可跨区购药,即同一城区内的药店仅为该区域内的小区提供服务。因此,初始连接关系为同一行政区内小区和药店间的全连接。每条连接边上的流量分配与该连接所对应的空间距离直接相关,流量随距离的增加而减小,递减速率由陡到缓,采用负指数函数对空间距离 d ij 与人流量 F ij bike 之间的定量关系进行建模如式（8）所示。
F ij bike = α e β d ij
式中：i为小区;j为药店; α 和 β 为参数。小区i与药店j之间对应的流量分配比例 R ij 计算方法如式（9）所示。
R ij = F ij bike ∑ j = 0 N F ij bike
式中：N表示为小区i提供服务的药店总数。

2.3 药店单服务台服务规则

城市内药店服务台一般都是单服务台,本文针对单服务台模式定义了如下规则：
（1）到达速率规则：顾客源是有限的,通过第2.1节可估算每个小区的购药人数。通过第2.2节可获得小区与药店间一对多的连接关系,以及小区购药流量在各条连接上的分配比例。依据排队论原理,假设顾客到达服务台的时间间隔服从负指数分布,顾客到达速率 λ 的确定方式如式（10）所示。
λ j = ∑ i = 0 n F i R ij T cycle × T operate
式中： λ j 为服务台j的顾客到达速率/（人/min）; F i 为小区i的购药流量/人; R ij 为小区i前往服务台j的流量比例; T cycle 为购药周期/d; T operate 为每日营业时长/min。
（2）排队规则：每个服务台排单队列,为队列长度设定上限,采取先到先服务的规则。
（3）服务时间：每个药店仅设单个服务台,所有药店并行服务,由于服务内容同质化,服务时长差异不大,因此将各顾客的服务速率 μ 均设为 0.2/（人/min）。

2.4 基于离散事件的购药过程仿真

采用基于离散事件的仿真方法对顾客购药排队过程进行仿真,涉及仿真实体、行为规则和仿真事件3种要素。其中,实体记录各方参与者的属性信息,行为规则是对实体行为过程的逻辑化表达,事件是能够引起实体状态发生改变的行为。本文将购药过程抽象为3种实体（顾客、服务台和队列）,6种行为规则,以及3种事件（等待、服务和流失）。
（1）仿真实体
定义1：将有购药需求的个人定义为顾客实体V见式（11）,实体属性包含顾客标识 I D visitor ,该顾客所属的小区标识 I D community ,目标药店标识 I D serve ,以及到达药店的时间 T arrive 。
V = { I D visitor , I D community , I D serve , T arrive }
定义2：将药店定义为服务台实体S见式（12）,其属性包含服务台标识 I D serve ,服务状态 S state 。
S = { I D serve , S state }
定义3：将队列定义为实体Q见式（13）,属性包含等候顾客的标识 I D visitor ,队列所属服务台标识 I D serve ,顾客加入队列的时间 T join 。
Q = { I D visito r , I D serve , T join }
（2）行为规则
依据现实情况,考虑了顾客成批到达的情形。顾客到达服务台后,依据新到顾客数量N,服务台状态 S state ,队列长度L,以及队列容量 L max 来决定顾客执行的行为。决策规则见表2,仿真流程如图2所示。
表2 顾客到达行为决策规则

Tab. 2 Behavioral decision rules upon customer arrival

组合规则 顾客行为
Sstate=0 且 N=1 被服务
Sstate=0 且 N>1 且 L+N<Lmax 被服务+排队
Sstate=0 且 N>1 且 L+N>Lmax 被服务+排队+离开
Sstate=1 且 L<Lmax 且 L+N<Lmax 排队
Sstate=1 且 L<Lmax 且 L+N>Lmax 排队+离开
Sstate=1 且 L=Lmax 离开
图2 顾客到达行为仿真流程

Fig. 2 Flow chart of customer behavior simulation

（3）仿真事件
定义4：将顾客加入等候队列的行为定义为等待事件 Even t wait 见式 ( 14 ) ,事件记录了顾客加入队列的时间 T join ,执行该行为的顾客标识 I D visitor ,以及所在服务台标识 I D serve 。
Even t wait = { T join , I D visitor , I D serve }
定义5：将顾客接受服务的行为定义为服务事件 Even t serve 见式 ( 15 ) ,事件记录了开始服务的时间 T serve ,接受服务的顾客标识 I D visitor ,提供服务的服务台标识 I D serve 。
Even t serve = { T serve , I D visitor , I D serve }
定义6：将顾客因等候人数达到上限而离开服务台的行为定义为流失事件 Even t lose 见式（16）,事件记录了顾客离开的时间 T lose ,离开的顾客标识 I D visitor ,相应的药店标识 I D serve 。
Even t lose = { T lose , I D visitor , I D serve }
在疫情防控期间,患者可通过社区代购、第三方物流或出门购药3种主要途径获取药物。从本文的建模逻辑来看,对药店的服务时长的消耗量,是区分不同购药方式的依据,这是由于药店的服务时长直接控制着仿真程序的起止时间,从而影响仿真结果和购药周期优化结果。上述3种方式虽然表现形式不同,但对药店服务时长的消耗量是相同的。因此,对于仿真求解的问题来说这3种方式本质上是相似的。为便于仿真的进行,本文假设患者均出门购药,来求解优化的购药周期。

2.5 最优购药周期求解流程

传染病防控下,购药周期的优化目标是在满足购药需求的前提下,一方面尽可能尽量减小购药频率,降低人群聚集引起的感染风险,即需要适当延长 T cycle ,另一方面充分利用药店的资源,方便患者购药,即需要酌情缩短 T cycle 。因此,这是一对互斥的优化目标,需要为 T cycle 找到折衷的取值。 T cycle 是否达到最优的判断依据是服务台的负载程度和资源闲置程度,一方面 T cycle 不能太小以致服务台负载过重,导致大量顾客无法被服务,另一方面 T cycle 不能太大导致服务台资源利用不充分。这里采用服务台平均未被服务人数 N unserved 这一指标反映服务台的负载和闲置情况。该指标由仿真结果统计得到,其中包含了服务台流失顾客数以及仿真结束后未被服务的顾客数。若 N unserved ≥ 1 表示服务台普遍存在需求不被满足的情况,相应的 T cycle 还需进一步优化,若 N unserved = 0 ,表明服务台普遍供过于求,存在资源闲置现象,因此将 N unserved < 1 作为 T cycle 是否最优的判断依据是合理的。最优购药周期的求解流程如下。
（1）确定 T cycle 初始值
为与排队论进行交叉验证,本文将排队论的稳态前提作为确定 T cycle 仿真初始值 T original_cycle 的依据,即令所有服务台均满足 λ ≤ μ ,求解结果为 T original_cycle =71。
（2）构造仿真实体
依据定义1,2,3分别构造实体V,S,Q。构造V需要依据总的购药人数和既定的 T cycle 确定日均购药人数,即V的数量,然后基于随机原则从顾客池中选取相应数量的顾客,并从中提取式11所需属性信息来构造实体V。构造S需要为每一个服务台生成唯一的标识 I D serve ,并将 S state 初始化为空闲状态。实体Q通过式（12）定义,仿真前需将其初始化为空,在仿真过程中当有顾客加入队列时,再向Q中实时添加。
（3）记录事件信息,更新实体状态
事件指引起实体状态发生改变的行为,在2.4节定义了3种事件,在仿真过程中需实时记录事件信息并更新相关实体的状态。当 V 到达 S 后,若 S 的队列长度已达 L max , V 会因等待时间成本过高而直接离开,此时触发 Even t lose 。若尚未达到 L max ,则 V 加入等候队列,同时在 Q 中进行注册。 S 完成当前服务解除占用后,在队列中等待的 V 获得服务机会,此时结束等待并触发 Even t wait ,同时依据 式（14）所示数据结构记录相关信息,该事件发生后,将相应 S 的 S state 更新为占用,并从 Q 中移除该 V 。当 S 完成对 V 的服务后,触发 Even t serve 并依据式（15）记录相关信息,同时将相应 S 的 S state 更新为空闲。
（4）二分法确定 T cycle 最优值
二分法是一种有序数列查找算法[18],易知随着 T cycle 的增大, N unserved 单调递减,因此 T cycle 可看作关于 N unserved 的有序数列。在优化过程中,以 T cycle （ 0 < T cycle < T original_cycle , T cycle ∈ Z ）作为查找对象,以 N unserved 作为查找依据。算法逻辑如下：
① 令 T a = 1 , T b = T original_cycle ;
② T i = T b - T a 2 ;
③ 计算 T cycle = T i 时的日均顾客服务量,运行仿真程序;
④ 统计 N unserved ,若 N unserved ≥ 1,令 T b = T i ,若 N unserved =0,令 T a = T i
⑤ 重复步骤②—步骤④,直至 T b - T a < ε （令 ε = 3 ）,输出结果 T a + T b 2 。

3 实验区概况及数据来源

3.1 实验区概况

武汉市位于湖北省东部,长江与汉江交汇处,下辖13个城区,其中,中心城区包括江汉区、硚口区、江岸区、汉阳区、武昌区、洪山区、青山区7个区,其余为远城区（图3）。武汉市居民小区数约为 15 000个,常住人口908.35万,流动人口超过500万。由于中心城区与远城区的实际情况存在差异,本文将研究区域限定在武汉市七大中心城区内。
图3 武汉市中心城区示意

Fig. 3 Schematic diagram of study area

3.2 数据来源

本文对武汉市封闭和复工阶段的慢性病药物保障情况进行建模,主要涉及以下数据集：
（1）武汉市建筑物面状数据来自网络开源数据（数据年份为2017年,下载网址为：http://bbs.3s001.com/thread-321007-1-1.html）,共计73 609条记录,数据范围涵盖整个武汉市区。其属性信息示例如表3所示。
表3 建筑物属性信息示例

Tab. 3 The data sample of building

FID 楼层数 建筑面积/m2
0 16 1267.8
1 16 18 485.4
2 16 10 595.3
… … …
（2）武汉市居民小区面状数据通过高德地图API获取（数据获取时间为2020年2月）,包含4908个小区,将小区与建筑物面积进行叠置分析,可估算小区的建筑物面积,已知武汉市人均住房面积为30 m2/人[19],可进一步估算小区尺度的人口数量,小区属性信息示例如表4所示。
表4 小区属性信息示例

Tab. 4 The data sample of community

FID 小区名称 人口数/人
1 027社区 5939
2 08经典 5619
3 122社区冶建花园东区 7567
… … …
（3）武汉市药店POI数据通过高德地图API获取（数据获取时间为2020年2月）,从中筛选出3937个连锁药店。本文假设仅考虑开放具有一定规模的连锁药店为市民提供购药服务,连锁药店筛选依据为药店名称出现3次以上,药店数据示例如表5所示。
表5 药店属性信息示例

Tab. 5 The data sample of drugstore

城区 名称 X Y 地址
洪山区 美佳康大药房（钢花南苑东南） … … 友谊大道1062号附近
汉阳区 中联大药房（江城明珠店） … … 四新地区管委会四新南路江城大道江城明珠
江汉区 武汉城镇职工医疗保险定点药店 … … 新湾五路与新湾路交叉口东150 m
（4）武汉市疫情期间购药相关的共享单车骑行订单数据源自摩拜单车,数据属性示例如表6所示。时间上,该数据跨度为10 d（2020年2月16日—2月26日）,日均骑行4.5万人次,空间上,完全覆盖了本文的研究区域,主要用于量化人流量随空间距离的变化规律。
表6 共享单车骑行订单属性信息示例

Tab. 6 The data sample of sharing bike orders

订单编号 用户ID 单车ID 起点X 起点Y 终点X 终点Y
275 29360203 1477421 … … … …
292 48853194 1477421 … … … …
317 65913653 1477639 … … … …

4 实证结果及分析

4.1 求解最优购药周期

4.1.1 各类患病人群数量估算结果
依据式（1）—式（7）对小区尺度的各类患病情况的出现概率及患病人数进行估算（表7）。武汉市慢性病患病率约为0.33473,该值与相关研究中的调查研究结果325.2‰[20]相近,患者总数约334.73万人。其中高血压患者（包括仅患有高血压和同时患有其他慢性病的情况）多达243.52万人,约占慢性病群体的72.76%,患病率次高的为糖尿病,约占患病人群总数的40.04%。同时患有3类疾病的人群占比约为4.3%。
表7 不同患病情况的出现概率及患者数量

Tab. 7 Probability and relevant number of patients under different diseases condition

患病情况 概率标记 概率取值 患病人数/万人
仅患有高血压 PA̅ 0.14921 149.21
仅患有糖尿病 PB̅ 0.06050 60.50
仅患有冠心病 PC̅ 0.01370 13.70
同时患有高血压和糖尿病 P(AB) 0.04200 42.00
同时患有高血压和冠心病 P(AC) 0.03780 37.80
同时患有糖尿病和冠心病 P(BC) 0.01701 17.01
同时患有高血压、
糖尿病和冠心病		 P(ABC) 0.01451 14.51
总计 0.33473 334.73
（1）药店与小区间的服务关系网络构建与流量分配结果
构建描述服务关系的拓扑时,依据疫情期间武汉市“不跨城区购药”的防控措施,原则上药店可以服务于同一城区内的任一小区,在拓扑结构上表达为药店与小区间的全连接（图4）。每一条连接上的流量大小与空间距离的远近紧密相关,空间距离增大对购药流量产生削减作用。本节基于疫情期间武汉市共享单车订单数据中与购药相关的记录对式（8）进行拟合,如图5所示,得到式中参数的取值为 α = 72.434 , β = - 0.635 。
图4 药店与小区间的服务关系网络拓扑

Fig. 4 Network of service relationship between drugstore and community

图5 购药流量与空间距离的拟合关系

Fig. 5 The fitting relation between the purchase flow and the spatial distance

4.1.2 购药最优值求解
T cycle 的初始值确定为71,因此,最优购药周期的初始取值范围为[1, 71]。基于二分法的 T cycle 优化求解过程如图6所示,经过4次迭代,最优值取值范围的首末值差值为2,达到迭代终止条件,此时最优值取值区间为[27, 29],因此最优值确定为28。
图6 购药周期最优值迭代查找过程

Fig. 6 Iterative process of searching for the optimal value of drug purchase cycle

4.2 仿真结果可靠性分析

为验证仿真逻辑的正确性和仿真结果的可靠性,本节将仿真方法与排队论对最优 T cycle 的求解结果进行对比。对比依据是描述系统状态的2项指标（队列长度L和等待时长W）,排队论求解 T cycle 需要令所有服务台达到稳态,解得 T cycle = 71 ,然后依据Little公式[21]计算指标值,如式（17）-式（18）所示。
L = λ μ - λ
W = L λ
运行仿真程序,对相同购药周期下的系统状态进行模拟（ T cycle = 71 ）,得到2项指标的仿真结果,将其与经典排队论Little公式的求解结果进行对比,如表8所示。
表8 基于排队论和事件仿真方法的队列特征值对比

Tab. 8 Queue eigenvalue comparison between queuing theory and event simulation method

平均队列长度L/人 人均等待时长W/min
Little公式 0.17 6.18
仿真结果 0.10 3.80
由表中数据可以看出, T cycle 为71时,2种方法得到的指标值均较小,平均队列长度趋近于0。对于仿真方法, L 指仿真周期内,每个服务台在每一仿真时刻排队人数的平均值。排队人数的取值为自然数（ LϵN ）,而仿真结果的L仅为0.10,意味着排队人数存在大量的0值,至少占到总样本数的90%。排队人数为0意味着顾客到达速率≤服务速率,由此可推断, T cycle 为71时,会导致一定程度的服务台资源闲置,还存在优化空间。2种方法求得的L仅相差0.07,在可接受的范围内,此外,2种方法的W差值与L的差值呈正相关,符合等待时间随队列长度增加而增加的这一规律。由此,仿真逻辑的正确性得到验证。

4.3 与排队论求解购药周期的对比分析

经典排队论方法通常以系统达到稳态为前提,再对相应的服务状态（L与W）进行分析[22]。但在现实场景中,各服务台顾客流量存在差异,若要满足稳态前提,需要令客流量最大的药店达到稳态来确定相应的购药策略,使得结果过于保守,导致小流量服务台利用率不高。相比于排队论,仿真方法无需以稳态为前提,并且能够动态地模拟服务台的等待能力（队列容量）对服务压力的缓冲作用,使得求解结果更加贴合实际。
由第3.3节和第3.4节可知,2种方法得到的最优 T cycle 分别为71和28。这里从供给侧和需求侧对2种方案的施行效果进行对比分析。从供给侧来看： T cycle 可调节服务台的日服务人数,进而影响服务台的忙碌程度,还可推算该周期内顾客的药物需求量,为药品限购量和药品备货量提供参考。从需求侧来看： T cycle 通过调节购药人流的聚集程度,影响着个人层面的购药时间成本。因此,通过服务台日服务人数、服务台繁忙时间占比,服务台药品日备货量,顾客等待时长等4项指标进行对比。统计尺度均为单服务台每天,结果如表9所示。
表9 2种最优购药周期的仿真结果对比

Tab. 9 Simulation results under two optimal drug purchase cycles

模型 购药周期/d 服务人数/人 繁忙时间占比/% 药品备货量/瓶或盒 顾客等待时长/min
排队论 71 86.68 90.29 38 3.85
仿真 28 92.61 96.47 94 8.55
相比之下,当 T cycle 取28,服务台的利用率提高了约6%,相应的药品备货量虽然增加近2.5倍但依然不超过100（瓶或盒）,相比疫情期间开放100个慢性病药店时慢性病药品日备货量逾万[23],本文开放3936个药店的店日均备货100（瓶或盒）,从药店人员流量控制和药物备货量来说是合理的。顾客平均等待时长约为8.55 min,调查研究显示,考虑顾客等待容忍度的最佳等待时间为8 min[24],与之相近。可见仿真方法确定的购药周期相比于经典排队论方法是更优的。下面从空间分析的角度对最优购药周期下小区和药店的分配关系和药店繁忙特性进行分析。
4.3.1 小区尺度的购药需求满足程度
需求未被满足的慢性病患者数量的空间分布如图7所示,每个数据点表示一个小区,数据点颜色取决于该小区购药需求未被满足的患者数（人）。依据图7中未满足患者数的分级标准,对小区数进行分级统计,结果如表10所示。研究区有3535个小区的购药需求完全得到满足,占到小区总数的93.7%。未满足的购药人数总量为1495人,约为总需求量的 0.4 ‰ ,总体来说,绝大多数的购药需求能够被满足。对于需求未满足的小区,待满足人数的最大值仅为26,其中取值为1-5的小区,约占69%。
图7 需求未被满足的慢性病患者数量的空间分布

Fig. 7 Spatial distribution of the number of chronic diseases with unmet needs

表10 基于未满足需求患者数的小区数量分级统计

Tab. 10 The number of communities under different level of unmet drug demands

城区 1~5/个 6~10/个 11~15/个 ≥16/个 小区数总计/个
东西湖区 17 1 3 1 22
汉阳区 17 3 2 8 30
洪山区 44 1 2 7 54
江岸区 26 2 0 7 35
江汉区 17 1 1 3 22
硚口区 12 0 3 12 27
青山区 7 0 0 2 9
武昌区 23 4 3 8 38
可以看出,需求未满足小区从中心向外逐渐减少,主要集中在长江沿岸地区,且西岸多于东岸。各城区中,武昌区未满足小区数最多,硚口区次之,主要是人口密度较大所致。东西湖区未满足小区数量最少,主要是由于该区小区总数较小,洪山区作为面积最大的城区,小区分布相较长江沿岸地区更为分散,因此并未出现大量小区需求不被满足的情况。
4.3.2 药店繁忙度的空间分布特征
药店繁忙度定义为其服务时长与营业总时长的比值,可用于描述药店的服务压力,换言之,药店的服务资源的利用率。繁忙度由低到高按相等间隔分为四级：空闲,正常,较繁忙,繁忙,研究区各药店繁忙度可视化如图8所示,各级繁忙度下药店的比例如表11所示。可见87.07%的药店繁忙度超过0.9,处于繁忙状态,空闲时间不足1 h,这意味着该限购周期下,药店需要做好轮换班工作,以应对较大的服务压力;空闲药店依然存在,,但这部分药店不足5%,表明药店的服务资源没有造成大的浪费这一方面印证了药店服务压力由于空间位置而产生分异的现象,另一方面从风险控制的角度来看,并非所有的药店都有开业的必要,在发生重大传染性公共卫生事件时,可以结合空间位置为药店赋予不同的效用值,优先开放效用值高的药店可以较好地兼顾需求的满足和风险的控制。
图8 药店繁忙度空间分布

Fig. 8 Distribution of busy level of drugstores

表11 不同繁忙度下的药店比例

Tab. 11 The proportion of drugstores under different busyness degree

繁忙度 药店比例/%
空闲(<0.3) 4.41
正常(0.3~0.6) 0.70
较繁忙(0.6~0.9) 7.82
繁忙(>0.9) 87.07

5 结论与讨论

5.1 结论

本文提出了传染病疫情防控下的市民慢性病药品购买周期优化方法,该方法考虑了空间距离对购药人流量的削减作用,通过复杂网络对购药人流在各药店间的分配比例进行建模,并进一步估算药店侧的顾客到达速率,以此实现排队论与离散事件仿真方法的耦合,以便对任意设定的购药周期的施行效果进行仿真。本文提出的方法不仅考虑了空间布局差异性对建模的影响,并且通过模型耦合,拓宽了经典排队论在系统稳态之外的适用性。基于本文提出的方法,以武汉市中心城区为例,求解了相应的最优的购药周期。主要结论如下：
（1）武汉市中心城区慢性病患者的最优购药周期为28 d。
（2）在同一购药周期下,本文提出的购药过程仿真方法与传统排队论方法得到的队列特征值（平均队列长度和人均等待时长）相近,从而验证了仿真逻辑的合理性。
（3）传统排队论方法求解得到的最优购药周期为71 d,相比之下,本文提出的方法所得到的方案（28 d）能够在合理的等待时长内（人均等待时长为8.55 min,接近可容忍的最佳等待时间8 min）,使患者的购药需求基本得到满足,同时可以在可接受的备货压力下（日均备货量不超过100（瓶或盒））,将服务台利用率提高近6%。

5.2 讨论

许多疾病可经空气传播而引起大流行,目前世界上41种主要传染病中,经空气传播的有14种,在各种传播中占首位,如流感就在全球范围内肆虐多次[25]。由于城市人口稠密,空气传播性疾病往往会在城市地区造成重大影响,对此,控制和减少聚集性购物活动是降低人群感染风险的有效策略,常采用的方法是限购。合理的限购策略能够在疫情防控的特殊时期,保质保量地满足城市居民的物资需求,保障市民的日常生活,维持社会的安定和正常运转。合理地制定限购策略需要对物资的需求、供给以及购买过程有较为深入的理解,因此,本文对限购策略的优化进行研究具有重要的实用价值和理论价值。
在本研究中,为了便于衡量购药周期对药店每日服务人次的调节作用,仅采用线性关系对其进行描述,即假设每天的购药人数是可控的,其取值为总购药人次在购药周期内的日平均值。该假设在现实生活中具有一定的可行性,比如疫情期间所采用的线上预约的方式,只需限制每日的预约人数即可达到控制购药人数的目的。但考虑到复工阶段,购药自由度更高,每天的购药人数会更多地取决于人的自主行为。在后续的研究中可结合有限理性[26]等影响自主决策的理论和方法对购药周期内的每天购药人数进行差异化建模,使得仿真效果更贴近实际情况。

References

Publishing order | Descend order by publishing year | Descend order by cited within
[1]
华春彬. 浅谈呼吸道传染病的特点及预防与控制[J]. 医学动物防制, 2012,28(3):333-334.

[ Hua C B. On the characteristics, prevention and control of respiratory tract infectious diseases[J]. Journal of Medical Pest Control, 2012,28(3):333-334. ]

[2]
徐田梅. 冬春季呼吸道传染病的预防与控制[J]. 中国卫生产业, 2012(8):180.

[ Xu T M. Prevention and control of respiratory tract infectious diseases in winter and spring[J]. China Health Industry, 2012(8):180. ]

[3]
中国日报. 有序推动复工复产.[EB/OL]. http://cn.chinadaily.com.cn/a/202002/23/html, 2020-02-23.

[ China Daily. Promote orderly resumption of work and production[EB/OL]. http://cn.chinadaily.com.cn/a/202002/23/WS5e51ea7ca3107bb6b57a1a10.html, 2020-02-23.

[4]
陈少红, 王建丽. 慢性病患者用药的依赖性探讨[J]. 中国临床医药研究杂志, 2003(109):11492-11493.

[ Chen S H, Wang J L. Discussion on drug dependence of chronic disease patients[J]. Chinese Journal of Clinical Research, 2003(109):11492-11493. ]

[5]
顾东风, 江河, 吴锡桂, 等. 中国成年人主要死亡原因及其危险因素[J]. 中国慢性病预防与控制, 2006,14(3):149-154.

[ Gu D F, Jiang H, Wu X G, et al. Leading causes of death and risk factors among Chinese adults[J]. Chinese Journal of Prevention and Control of Chronic, 2006,14(3):149-154. ]

[6]
邓方安, 刘三阳. 市场供求平衡中的模糊模型[J]. 西安电子科技大学学报, 2002,29(2):284-288.

[ Deng F A, Liu S Y. The fuzzy model of equilibrium of supply and demand in markets[J]. Journal of Xidian University, 2002,29(2):284-288. ]

[7]
林晗. 煤炭市场供需平衡分析及对策研究[D]. 大连:大连海事大学, 2006.

[ Lin H. Analysis on the balance of coal supply and demand and the research on countermeasures[D]. Dalian: Dalian Maritime University, 2006. ]

[8]
孙静春, 吕丁, 袁治平, 等. 燃料价格对城市车用天然气供需平衡的仿真分析[J]. 系统工程理论与实践, 2008,28(2):43-51.

[ Sun J C, Lv D, Yuan Z P, et al. Simulation analysis of fuel price to supply and demand balance on automobile-used natural gas in city[J]. Systems Engineering-Theory & Practice, 2008,28(2):43-51. ]

[9]
李朴, 欧文斌, 叶苓, 等. 基于排队论模型的口腔科门诊医师配置量化分析[J]. 医学研究生学报, 2014,27(8):863-865.

[ Li P, Ou W B, Ye L, et al. A quantitative analysis of outpatient physician allocation in stomatology department based on queuing theory model[J]. Journal of Medical Postgraduates, 2014,27(8):863-865. ]

[10]
朱志良, 邱媛源, 李丹程, 等. 一种Web服务复杂网络的构建方法[J]. 小型微型计算机系统, 2012,33(2):199-205.

[ Zhu Z L, Qiu Y Y, Li D C, et al. A method of constructing complex network of Web services[J]. Journal of Chinese Computer Systems, 2012,33(2):199-205. ]

[11]
刘国仗, 于汇民. 高血压合并糖尿病的治疗[J]. 中国心血管杂志, 2002,7(2):84-89.

[ Liu G Z, Yu H M. Treatment of hypertension with diabetes mellitus[J]. Chinese Journal of Cardiovascular Medicine, 2002,7(2):84-89. ]

[12]
孙言凤, 李静娜, 吴晓旻, 等. 武汉市某地区居民健康状况及危险因素调查[J]. 医学动物防制, 2014,30(2):183-185.

[ Sun Y F, Li J N, Wu X W, et al. Investigation on the health status and risk factors of residents in a district of wuhan[J]. Journal of Medical Pest Control, 2014,30(2):183-185. ]

[13]
金春花, 李连喜, 李梅芳, 等. 新诊断2型糖尿病患者视网膜微血管异常的临床特征及危险因素研究[J]. 中国全科医学, 2015,18(5):493-497.

[ Jin C H, Li L X, Li M F, et al. Clinical characteristics and risk factors of newly diagnosed retinal microvascular abnormalities in type 2 diabetes mellitus[J]. Chinese General Practice, 2015,18(5):493-497. ]

[14]
谭晓云, 李宁燕, 郭源, 等. 社区居民生活方式相关疾病及相关因素调查分析[J]. 中国临床保健杂志, 2008,11(1):13-15.

[ Tan X Y, Li N Y, Guo Y, et al. Investigation and analysis of lifestyle related diseases and related factors of community residents[J]. Chinese Journal of Clinical Healthcare, 2008,11(1):13-15. ]

[15]
陈国伟. 高血压与糖尿病[J]. 中国实用内科杂志, 2002,22(4):199-200.

[ Chen G W. Hypertension and diabetes[J]. Chinese Journal of Practical Internal Medicine, 2002,22(4):199-200. ]

[16]
赵莉莉. 老年冠心病合并高血压患者的临床护理体会[J]. 中国药物与临床, 2019,19(10):138-140.

[ Chen L L. Clinical nursing experience of elderly patients with coronary heart disease and hypertension[J]. Chinese Remedies & Clinics, 2019,19(10):138-140. ]

[17]
张慧. 冠心病合并糖代谢异常中医证型随访研究[D]. 广州:广州中医药大学, 2007.

[ Zhang H. Follow-up study on syndrome type of coronary heart disease with abnormal glucose metabolism[D]. Guangzhou: Traditional Chinese Medicine University of Guangzhou, 2007. ]

[18]
王海涛, 朱洪. 改进的二分法查找[J]. 计算机工程, 2006,32(10):60-62.

[ Wang H T, Zhu H. Improved binary search[J]. Computer Engineering, 2006,32(10):60-62. ]

[19]
龚恰. 我国城镇住房保障制度存在的问题及建议[J]. 环渤海经济瞭望, 2010(8):19-20.

[ Gong Q. Problems and Suggestions of urban housing security system in China[J]. Economic Outlook the Bohai Sea, 2010(8):19-20. ]

[20]
秦江海, 张艳春, 张丽芳, 等. 典型城市居民慢性病患病率及患者疾病负担分析[J]. 中国公共卫生, 2014,30(1):5-7.

[ Qin J H, Zhang Y C, Zhang L F, et al. Prevalence of non-communicable diseases and economic burden of patients in 8 typical cities[J]. Chinese Journal of Public Health, 2014,30(1):5-7. ]

[21]
孙健, 丁日佳, 陈艳艳. M/M/c型与M/M/1型排队系统对比仿真[J]. 北京工业大学学报, 2016,42(9):1324-1331.

[ Sun J, Ding R J, Chen Y Y. M/M/c and M/M/1 queuing system comparative simulation[J]. Journal of Beijing University of Technology, 2016,42(9):1324-1331. ]

[22]
赵彤宇, 张乃通. 基于事件触发的通信业务量仿真方法[J]. 通信技术, 2002(11):70-72.

[ Zhao Y T, Zhang N T. A traffic simulation method based on event trigger[J]. Communication Technology, 2002(11):70-72. ]

[23]
新华社. 探访武汉慢病重症药店:取药员每日人均拿药3万盒[EB/OL]. https://www.sohu.com/a/376529356_267106, 2020-02-28.

[ the Xinhua News Agency. Visit a chronic and severe drug store in wuhan: The average dispenser takes 30, 000 boxes of medicine per day[EB/OL]. https://www.sohu.com/a/376529356_267106, 2020-02-28.]

[24]
李华敏, 张辉. 顾客等待容忍度与银行排队服务系统的优化[J]. 金融论坛, 2011,16(2):29-34.

[ Li H M, Zhang H. Customer waiting tolerance and optimization of bank queuing service system[J]. Finance Forum, 2011,16(2):29-34. ]

[25]
谢雍宁. 苍术艾叶烟熏预防呼吸道传染病[J]. 医学信息, 2011,24(11):346.

[ Xie Y N. Prevention of respiratory infectious diseases by smoky atractylodes and artemisiaargyi[J]. Medicine Information, 2011,24(11):346. ]

[26]
仪垂林, 张力美. 有限理性、情绪与决策[J]. 统计与决策, 2005(11):48-50.

[ Yi C L, Zhang L M. Bounded rationality, emotions, and decision making[J]. Statistics & Decision, 2005(11):48-50. ]

Options
Outlines

/

〈 〉